这里，利用被称为诺伊曼问题的解析解来验证凝固。 这个固化问题的分析解决方案 被胡等人[1] 和 Kawahara等人[2] 等人使用。
实验描述
将最初为液态的板坯从一侧冷却至熔点以下。 可以获得温度分布和熔体前沿位置的解析解，以便与模拟数据进行比较。 接口位置可以从以下位置获得：
$x(t) =2Q \sqrt{\alpha t}~~~~~~~(1)$$
Q 是通过一个取决于材料属性的超越方程得出的，对于本次比较中使用的材料属性，其结果为 1.19 α是热扩散率，t是时间。 固相温度的解析解为
$$T_s(x, t) = T_w + (T_m - T_w) * \frac{erf(x / (2.0 * \sqrt{\alpha_s *t)})} { erf(Q)}~~~~~~(2)$$
对于液相
$ T_l(x, t) = T_{inf} + (T_m - T_{inf}) * \frac{erfc(x / (2.0 * \sqrt{\alpha_l * t}))} {erfc(Q)}~~~~~~(3)$
热物理性质总结在表中。
| 参数 | 值 |
|---|---|
| 加热电压 | 400 W/(m*K) |
| 密度 | 1000.0 kg/m3 |
| 比热容 | 1000.0 J/(kg*K) |
| 潜热 | 200000.0 J/m3 |
| 左墙 | 173.15 K |
| 右墙 | 283.15 K |
| 初始熔点 | 283.15 K |
模拟设置描述
设置二维模拟，其中一个粒子区域表示熔体，两个多边形区域表示左壁和右壁 粒子半径设置为0.001米，忽略重力的影响。 下图显示了初始粒子区域的几何设置。 温度样本沿 x 轴放置，以便与分析结果进行比较 液体的初始温度设置为 283.15 K。左壁面的温度恒定为 173.15 K。此壁面用于将初始液体冷却至凝固点以下 右壁的温度设置为与初始流体温度相同。
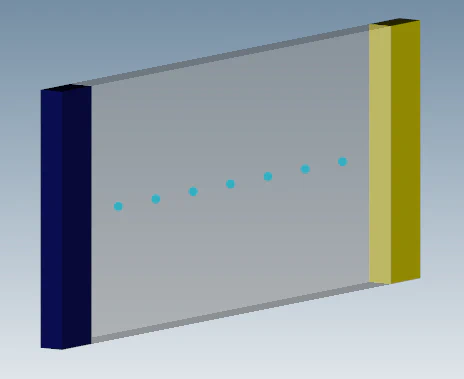
https://shoncloud.com/images/cash studies/sonDy_Neumann/Geometry.png does not exist模拟结果与实验的比较
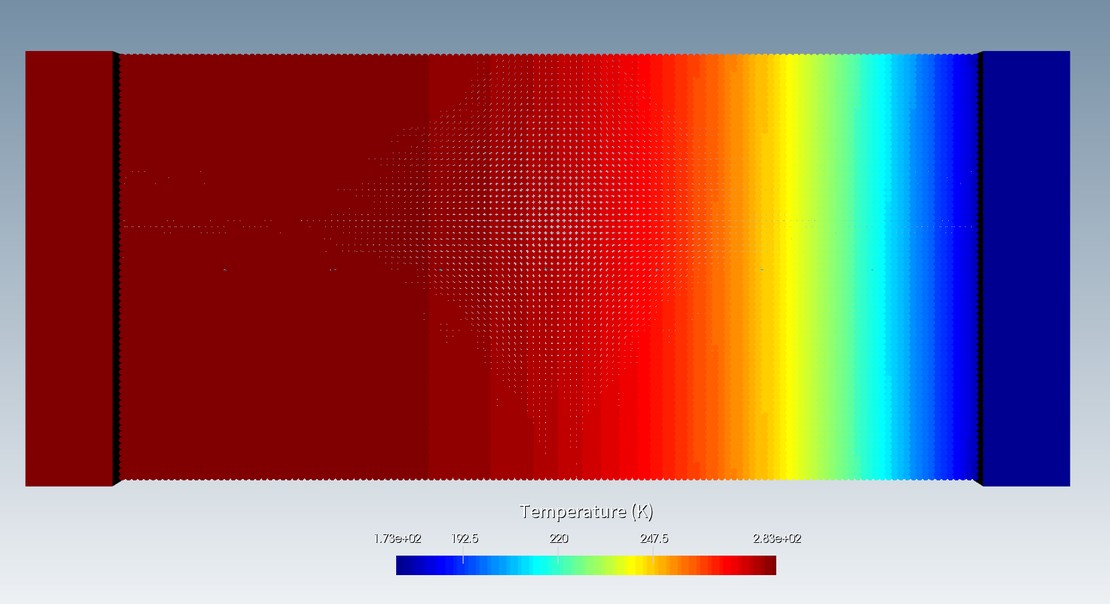
时间点t=4s时温度分布的模拟结果如下图所示 左壁和右壁保持恒定的初始温度。 液体从左到右被冷却下来。
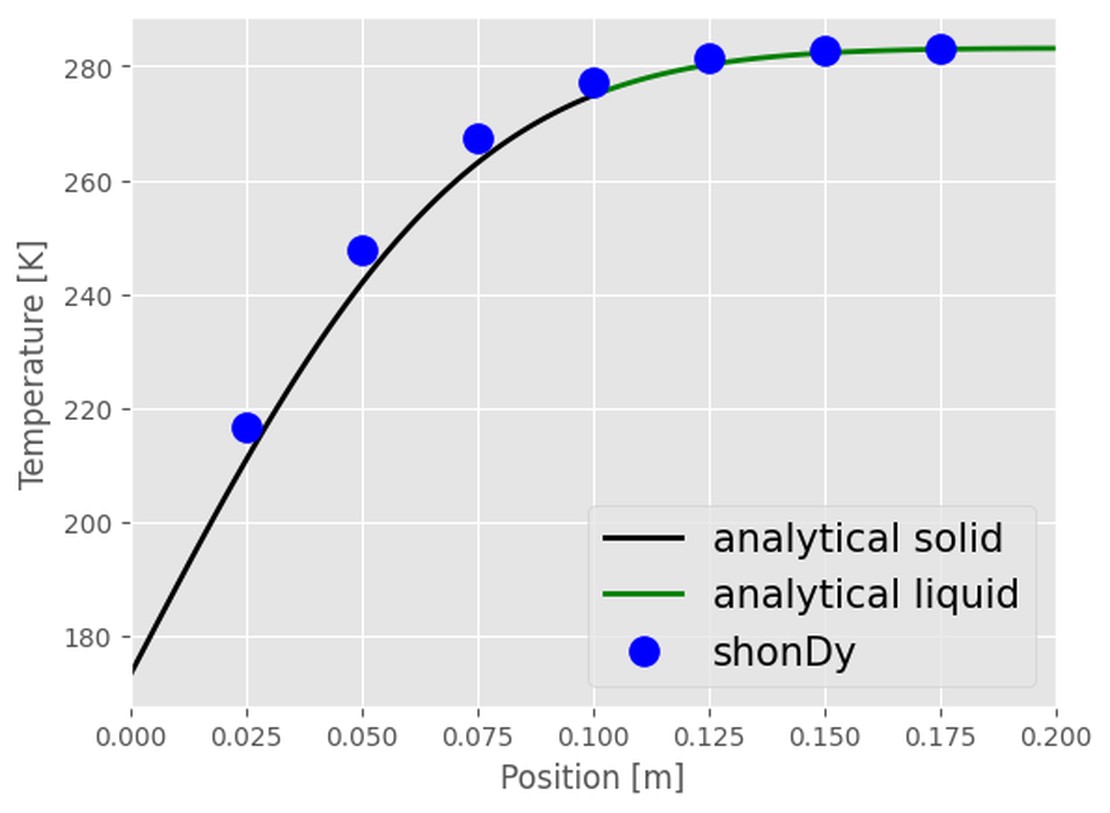
https://shoncloud.com/images/cash studies/sonDy_Neumann/Geometry.png does not exist该图显示了时间 t = 4 s 时的分析结果和模拟结果的比较。 在左图中，黑线表示固体温度的分析结果（见公式 (2)），而绿线表示液相的温度分布（见公式3） 在左图中，黑线表示固体温度的分析结果（见公式 (2)），而绿线表示液相的温度分布（见公式 总体而言，分析结果和模拟结果具有很好的一致性。
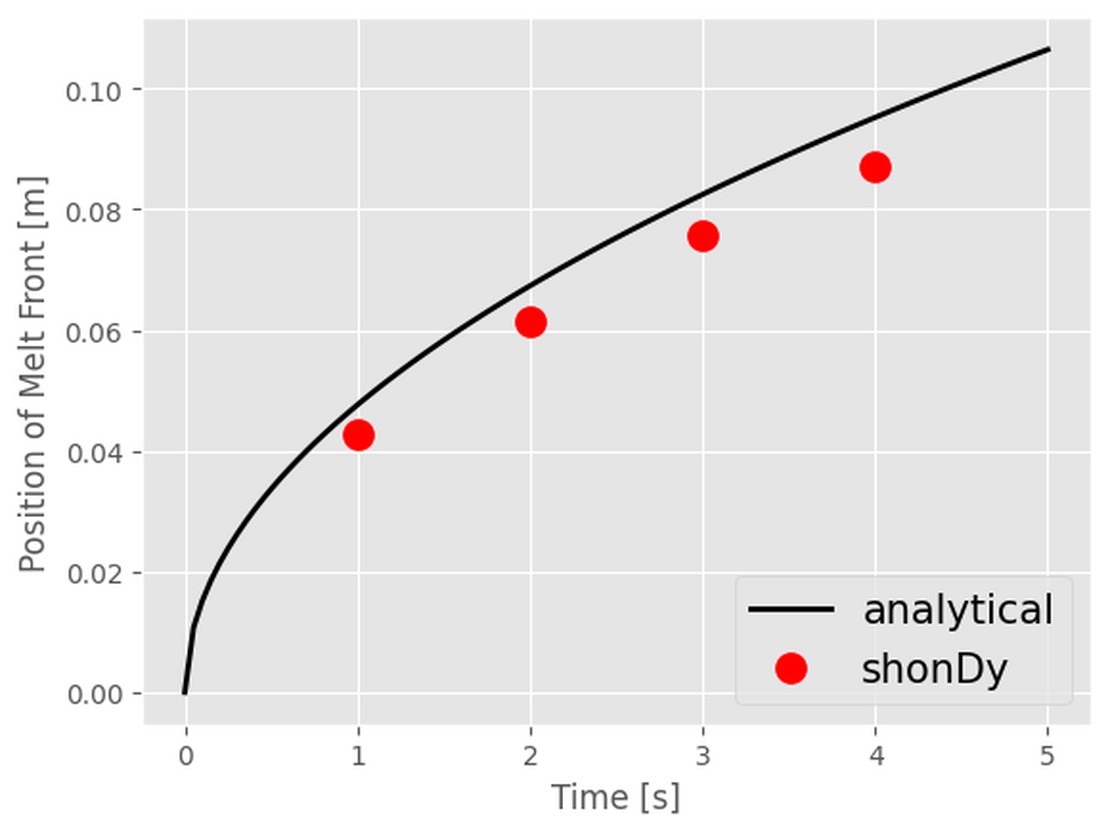
右图比较了熔体前沿的进展 使用公式（1）计算分析结果（黑线），而红点表示模拟结果。
[1] H. Hu 和 S. A. Argyropoulos, “Mathematical modelling of solidification and melting: a review”, Modelling and Simulation in Materials Science and Engineering, vol. [2] T. Kawahara 和 Y. Oka，“通过移动粒子半隐式方法研究堆芯外熔融物凝固行为”，核科学与技术杂志，第 35 卷，第 2879-2895 页。
49，第12期，第1156-1164页，2012年。 49, no. 12, pp. 1156-1164, 2012.
